|
A VISUAL NOTATION FOR RATIONAL NUMBERS MOD 1 JULIE TOLMIE
Name: Julie A. Tolmie, Visual Mathematician, (b. Brisbane, Qld., Australia, 1958). Address: (pending immigration) TechBC (Technical University of British Columbia), 2400 Surrey Place, 10153 King George Highway, Surrey, BC, CANADA E-mail: jatolmie@ozemail.com.au Fields of interest: Mathematical visualisation; Visual notation (2D maps, 3D navigable environments); History and philosophy of the scientific image (abstract film and abstract dance, early history of Western musical notation, multimedia as total theatre).
Publications and/or Exhibitions: Tolmie, J.A. (2000) Visualisation, Navigation and Mathematical Perception: A Visual Notation for Rational Numbers Mod 1, [Ph.D. Dissertation], Canberra: Australian National University, 4CDs, 300 animations. Tolmie, J.A. (2001) Phase space à côté, In: Intersections of Art and Science, [Exhibition], Sydney: Ivan Dougherty Gallery, COFA, UNSW, [Digital image and animation].
Abstract: This paper presents a methodology for
the construction of visual notation. Visual primitives are chosen. New
visual objects are constructed from them. The formalism assumes that once
a visual object has been stated, it exists and can be drawn on for use
in superposition with, or substitution of, other visual objects. The demonstrated
example is rational numbers (or fractions). The torus, with its two directions
of rotational symmetry, is used as a phase space; its longitudinal cycle
for the denominator; its meridian cycle for the numerator. This results
in three- dimensional navigable objects, two of which are discussed. Dominant
visual structures are observed in both of these objects. An application
of the second visual object orders the bud size of the buds of the main
cardioid of the Mandelbrot boundary.
1 VISUAL STRUCTURES IN LIEU OF TEXT (A visual abstract is provided at http://www.ozemail.com.au/~jatolmie/isis0.html.) This work would not exist had it been confined by linear language forms. A visual formalism is introduced whereby visual objects constructed from previous visual objects and visual primitives substitute for the usual text-based definitions. The challenge was to put rational numbers on stage by choreographing their movements to reveal, or offer insight into, their mathematical structure. To do this involved the questioning of many unwritten conventions. For example, mathematics as a text-based form inherits conventions from natural language (Nordon 1994). As a spatial form, it will inherit conventions from film, theatre, dance, photography, and animation. What are the devices used to punctuate these spaces, and why do they work? (McAuley 1987, Herbison-Evans 1988, Barthes 1964, 1970, 1973, Svoboda 1993, Le Grice 1977.) In text, mathematical symbols are separated by brackets,
commas, dots, operation signs, arrows, etc, with a convention to proceed
from left to right. In visual space, visual objects are frequently interwoven.
Preferred viewpoints and motions do not yet exist. They are established
experimentally, and are to some extent dependent on the type of mathematics
notated, and the type of notation used to notate it. While the initial
example chosen is geometric in nature (rational numbers mod 1 encoded
spatially using two coordinates of rotational symmetry), it is the formalisation
of pattern and visual objects, and their recognition, which could be applied
in a more abstract context.
2 RATIONAL NUMBERS AS CYCLIC CONFIGURATIONS
Rational numbers mod 1 are represented abstractly
as equivalence classes of pairs (p, q). But they can also
be visualized as parts of a cycle. We would like to use this second notion
to create visual symbols for them. Firstly, the denominator of a rational
number is encoded as radial direction (in the horizontal plane). Then,
using cyclic motion and a discrete map of {1, 2, 3, … 37} into the RGBcolour
space, the numerator is encoded as cyclic permutations of colour coded
dots. These configurations are placed in the appropriate meridian slices
(in radial vertical planes). The result is a three dimensional navigable
object contained in a torus. In three dimensions, colour alignments and
relative distributions of elements are the most striking aspects to the
space. In two dimensions, sequences of rational numbers are viewed as abstract
animation. Dominant visual structures are perceived dynamically. Radial
directions behave simultaneously as sources and sinks. The subpatterns
of convergence are analyzed by defining each radial direction as a sequence
in time. A particular radial direction either appears or does not appear
in an individual frame of the animation. Tracing the subpatterns backwards
reveals the Farey tree structure of the rational numbers mod 1.
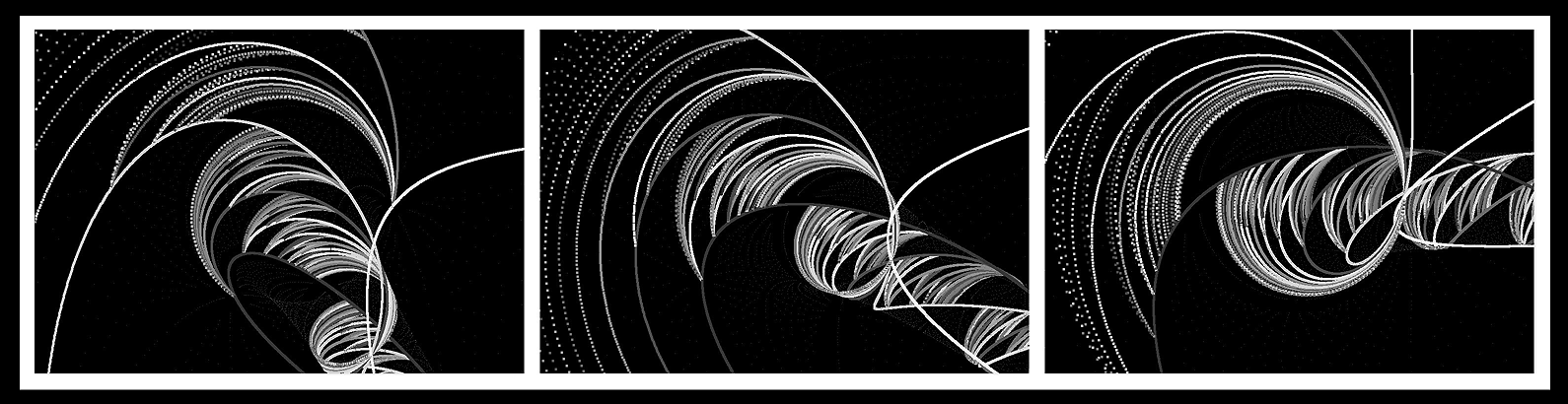
3 RATIONAL NUMBERS AS FAREY CURVE SEGMENTS The Farey tree is a binary tree construction of the rational numbers. (see Bogomolny http://www.cut-the-knot.com/blue/Farey.html). To introduce rotational symmetry, the Farey tree is made circular and embedded in the longitudinal plane of the torus. Individual rationals are then defined as curve segments which span the longitudinal region bounded by its Farey parents. These curve segments are cut from curves which wind around the torus at a constant integer velocity (integer velocity one parameter subgroups of the torus as a Lie group). A three dimensional navigable object is made.
The dominant visual structures are "fan" like objects
which emanate from rational lattice points above and below the outer longitude
of the torus. The rational numbers mod 1 are then ordered by these
fans, providing insight into their tidal interweaving.
4 APPLICATION TO MANDELBROT SET –
There is an immediate application: The buds of the main cardioid of the Mandelbrot boundary can be put into one to one correspondence with the rational numbers mod 1. We "forget" its continuous structure. We are only interested in the ordering of the buds, by size, in sequences of buds, and in the interweaving of these sequences. It turns out that the above ordering of rational numbers mod 1 by fans induces a natural ordering on the bud size of sequences of buds of the main cardioid of the Mandelbrot boundary. A visual notation is created by using a two dimensional
fan to denote a three dimensional fan. The circular Farey tree is embedded
in the unit circle and fans are attached to its vertices with endpoints
the rational points on the circle. A single vertex in the tree now represents
an infinite sequence of rational numbers. In this way, the internal Farey
trees acts as an indexing set for the tiling of the Mandelbrot boundary
by infinite sequences of buds (fans). In other words, the interwoven extended
structure on the Mandelbrot boundary is disentangled by mapping it to distinct
initial points in the Farey tree. In this representation, individual buds
do not exist. The primitive unit is the sequence of buds. As such, the
fans on the torus provide a three dimensional phase space for viewing the
discrete visual structure of the Mandelbrot boundary. (See on the front
page of this Journal.)
References Barthes, R. (1982) L’Obvie et l’Obtus Essais Critiques III, Paris: Éditions de Seuil, [Rhétorique de l’image (1964) 25-42, Le troisième sens (1970) 43-61, Diderot, Brecht, Eisenstein (1973) 86-93]. Douady, A. (1986) Itération de polynomes complexes, In: Courier du CNRS, Images des Mathématiques, Supplement au no. 62, 143-154. Le Grice, M. (1977) Abstract Film and Beyond, London: Studio Vista. McAuley, G. (1987) Exploring the paradoxes: On comparing film and theatre, In: Bell,P, and Hanet, K., eds., Film, TV, and the Popular, Special Issue Continuum: The Australian Journal of Media and Culture, Vol. 1, No.2, 44-55; also http://www.mcc.murdoch.edu.au/ReadingRoom/1.2/McAuley.html Moholy-Nagy, L. (1961) Theatre, circus, variety, In: Kirby, E.T., ed., Total Theatre: A Critical Anthology, New York: E.P. Dutton and Co., 114-124. Nordon, D. (1994) Mathématiques, forme littéraire, In: Cahiers, Art et Science, No. 1, Bordeaux: Université Bordeaux1 and Éditions Confluences, 105-123. Onishchik, A.L. (1993) Lie Groups and Lie Algebras, Berlin: Springer-Verlag. Prampolini, E. (1915) Futurist scenography, In: Kirby, E.T., ed., Total Theatre: A Critical Anthology, New York: E.P. Dutton and Co. Shubnikov, A.V. and Koptsik, V.A. (1974) Symmetry in Science and Art, New York: Plenum Press, [Russian text published for the Institute of Crystallography of the Academy of Sciences of the U.S.S.R., 1972]. Svoboda, J. (1993) The Secret of Theatrical Space, Edited and translated by Burian, J.M., New York: Applause Theatre Books.
|