|
LAPE'S HINGED DODECAHEDRON
WILLIAM S. HUFF
Name: William
S. Huff, Architect and educator (b. Pittsburgh, Pa., U.S.A., 1927). Professor
Emeritus, State University of New York at Buffalo 1326 Murray Avenue, Pittsburgh,
PA 15217
Address: Department
of Architecture, State University of New York at Buffalo, Buffalo, NY,
14214, U.S.A.
E-mail: wshuff@earthlink.net
Fields of interest: Architectural
design, basic design (including symmetry, theory of structure, topology,
Gestalt principles, color perception, functional application of color).
Publications: (2000)
Ordering Disorder after K. L. Wolf, in: Forma, vol. 15, Tokyo: KTK
Scientific Publishers, 41-47; (1996) The Programmed Design: Toward Hidden
Symmetries, in: [Abstract] Mathematics and Art: Second International
Conference "Nonlinear World," Moscow: Moscow State University, 67;
(1996) The Landscape Handscroll and the Parquet Deformation, in: Katachi
U Symmetry, Tokyo: Springer-Verlag, 307-314; (1995) The Programmed
Design: Probing the Discernibility of Properties of Symmetry, in: [Abstract]
Symmetry: Culture and Science, vol. 6, Budapest: ISIS-Symmetry,
254-257; (1975) 2, (1977) 3, (1967) 4, (1971) 5, (1970) 6, Symmetry:
an appreciation of its presence in man’s consciousness. Pittsburgh.
Abstract: The
trisectioning of the cube into congruent parts is one of several three-dimensional
assignment of my formative design studio that has been intermittently investigated
with my students for nearly 30 years. It grew out of a wider pedagogic
challenge: the sectioning of the cube into any number of congruent parts
and, even, the sectioning of other regular and semi-regular solids in such
wise.
1. Trisectioning of the cube
and the Rhombic Dodecahedron
A remarkable design from the unpredictable
pool of student creativity (in this case, from John Lape) came out of the
early phase of this studio task: a rhombic dodecahedron, sectioned into
24 congruent, non-regular, superposable tetrahedra. The art of this artifice—which,
arranged into 12 square-based, split pyramids, can lie flat on a plane
(Fig. 1)—is derived from the fashion in which the 24 parts are hinged together;
for it is most remarkable that there are two strikingly different ways
to assemble this hinged wizardry into a rhombic dodecahedral body. One
may be called the wrapped cube; the other, the radiant
pentahedra. A telling sign of this dualism lies in the recognition
that, in one assemblage, a joint cuts across the short diagonal of each
rhombic face and, in the other, a joint cuts across the long diagonal of
the rhombic face.
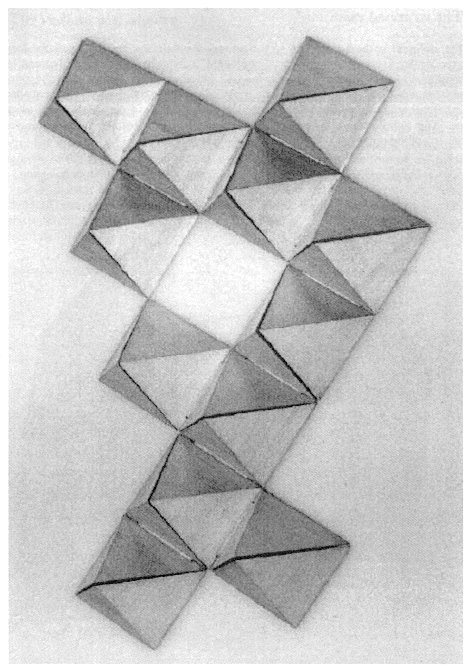
Figure 1.
2. The wrapped cube
At the midpoint in the assemblage
of the wrapped cube (Fig. 2), with one cube formed, the remainder
is easily plied into a conjoined twin cube. The assembler can, then, dissemble
either of the cubes and wrap the free dangling pieces around the still
assembled cube (somewhat like a hull fits around a nut) and complete the
rhombic dodecahedron—the one with unconnected butt joints running across
the short diagonals of the rhombic faces. (This vividly suggests how the
rhombic dodecahedron belongs to the cubic system of the crystallographer.)
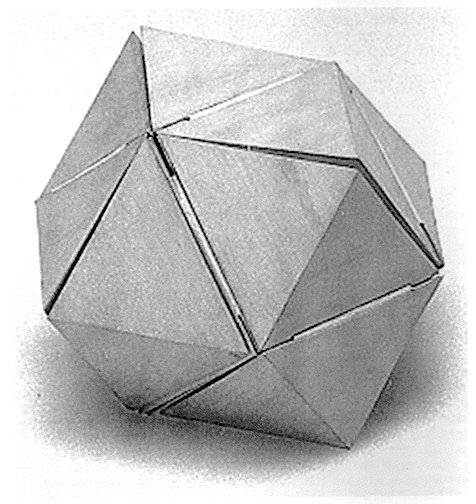
Figure 2
3. The radiant pentahedra
Before attempting the more difficult
feat that is met in the assemblage of the radiant pentahedra (Fig.
3), it does well to return the hinged configuration to the planar layout.
By pivoting two elemental units on the hinge that pairs them into a square-based,
split pyramid, a rhombic-based, split pyramid is formed; and as one after
another of these are in the like manner paired, the whole configuration
automatically begins to pull together (the apices of all 12 pyramids meeting
at the body’s center) into the alternatively assembled rhombic dodecahedron,
which displays hinged joints running across the long diagonals of the rhombic
faces. It is seen in the planar layout that four of the pyramids are connected
at their basal edges to three other pyramids, two are connected to two,
and six are connected to one. The six singly connected, square-based pyramids,
being re-paired into rhombic-based pyramids, will flip-flop into alternate
positions, as this version of the assembled dodecahedron is taking shape;
and an incorrect positioning of any one of them will throw the assemblage
out of kilter.
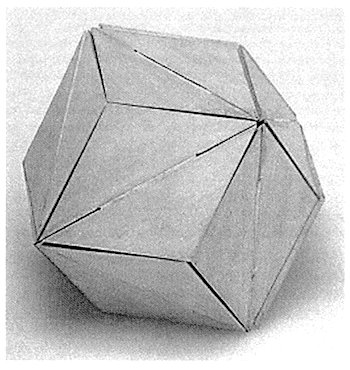
Figure 3
NEXT
HOME
|